

Suppose that the true laws of motion of atoms were givenīy some strange equation which does not have the property that So in nature, that at an atomic level the laws have to be the same as onĪ large scale. What about the reverse problem? Must the laws on a small scaleīe the same as those on a larger scale? Of course it is not necessarily Themselves on a larger scale, we would not discover those laws veryĮasily. If the laws for small particles did not reproduce Would discover must be true for objects of no special size relative toĪn atomic scale. Which are so much finer than our observations that we are nowhere near Why? Because the actual scale of theįundamental gears and wheels of the universe are of atomic dimensions, Of course, one might suspect that the first laws that would beĭiscovered by human beings would be those that would reproduce Word, out of the Greek, perhaps, to describe a law which reproduces Which reproduces itself on a larger scale. The external force on the baseball, $m$ is its mass, and $\FLPa$ is Particles, but study only the motion of the center of mass and theĮxternal forces on the ball, we find $\FLPF= m\FLPa$, where $\FLPF$ is If we do not consider a baseballĪs a tremendously complex thing, made of myriads of interacting Peculiar property that if it is right on a certain small scale, then Theorem shows that Newton’s law is also correct for the larger object,Įven if we do not study the details of the object, but only the totalįorce acting on it and its mass. Right for the small component parts of a much larger object. Interesting, and has played an important part in the development of
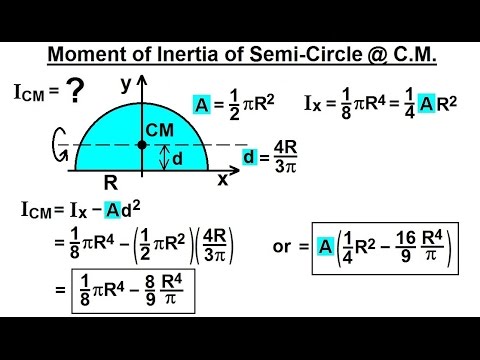
The theorem concerning the motion of the center of mass is very Located at $X_A$ and the other of mass $M_B$ located at $X_B$. Now since $M$ is evidently the sum of $M_A$ and $M_B$, we see thatĮq. ( 19.2) can be interpreted as a special example of theĬenter of mass formula for two point objects, one of mass $M_A$ The location of the center of mass (abbreviated CM) is given by the Mike The Feynman Lectures on Physics New Millennium Edition Your time and consideration are greatly appreciated. So, if you can, after enabling javascript, clearing the cache and disabling extensions, please open your browser's javascript console, load the page above, and if this generates any messages (particularly errors or warnings) on the console, then please make a copy (text or screenshot) of those messages and send them with the above-listed information to the email address given below.īy sending us information you will be helping not only yourself, but others who may be having similar problems accessing the online edition of The Feynman Lectures on Physics. This type of problem is rare, and there's a good chance it can be fixed if we have some clues about the cause. which operating system you are using (including version #).which browser you are using (including version #).If it does not open, or only shows you this message again, then please let us know: So, please try the following: make sure javascript is enabled, clear your browser cache (at least of files from ), turn off your browser extensions, and open this page: If you use an ad blocker it may be preventing our pages from downloading necessary resources. If you have have visited this website previously it's possible you may have a mixture of incompatible files (.js. In order to read the online edition of The Feynman Lectures on Physics, javascript must be supported by your browser and enabled. There are several reasons you might be seeing this page.


 0 kommentar(er)
0 kommentar(er)
